Black Body Radiation

In practice no material has been found to absorb all incoming radiation, but carbon in its graphite form absorbs all but about 3%. It is also a perfect emitter of radiation. At a particular temperature the black body would emit the maximum amount of energy possible for that temperature. This value is known as the black body radiation. It would emit at every wavelength of light as it must be able to absorb every wavelength to be sure of absorbing all incoming radiation. The maximum wavelength emitted by a black body radiator is infinite. It also emits a definite amount of energy at each wavelength for a particular temperature, so standard black body radiation curves can be drawn for each temperature, showing the energy radiated at each wavelength. All objects emit radiation above absolute zero.
.
Some Examples:
Objects at around room temperature emit mainly infra-red radiation (l» 10mm) which is invisible. The sun emits most of its radiation at visible wavelengths, particularly yellow (l » 0.5mm). A simple example of a black body radiator is the furnace. If there is a small hole in the door of the furnace heat energy can enter from the outside. Inside the furnace this is absorbed by the inside walls. The walls are very hot and are also emitting thermal radiation. This may be absorbed by another part of the furnace wall or it may escape through the whole in the door. This radiation that escapes may contain any wavelength. The furnace is in equilibrium as when it absorbs some radiation it emits some to make up for this and eventually a small amount of this emitted radiation may escape to compensate for the radiation that entered through the hole. Stars are also approximate black body radiators. Most of the light directed at a star is absorbed. It is therefore capable of absorbing all wavelengths of electromagnetic radiation, so is also capable of emitting all wavelengths of electromagnetic radiation. Most approximate blackbodies are solids but stars are an exception because the gas particles in them are so dense they are capable of absorbing the majority of the radiant energy.

The black body radiation curve (Fig1) shows that the black body does radiate energy at every wavelength. The curve gets infinitely close to the x-axis but never touches it. The curve touches at infinite wavelength. It also shows that the black body emits at a peak wavelength, at which most of the radiant energy is emitted. At 5000K the peak wavelength is about 5x10^-7m (500nm) which is in the visible light region, in the yellow-green section. At each temperature the black body emits a standard amount of energy. This is represented by the area under the curve.
 
 

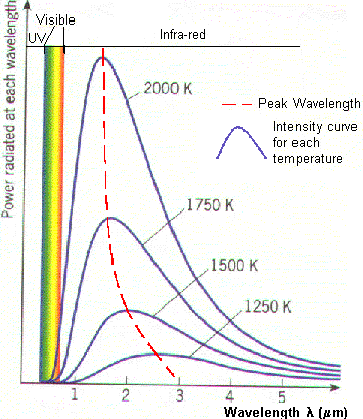This graph shows how the black body radiation curves change at various temperatures. These all have their peak wavelengths in the infra-red part of the spectrum as they are at a lower temperature than the previous graph.
The graph shows:
.

P = Power radiated  in W (J/s)   s = Stefan's Constant 5.67 x 10-8 W m-2 K-4  A = Surface area of body (m²)  T = Temperature of body (K)

Therefore the Power radiated is proportional to T⁴ for an identical body which explains why the area under the black body curves (the total energy) increases so much for a relatively small increase in temperature.
 

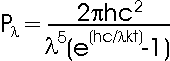Pl=Power per m² area per m wavelength  h = Planck's constant (6.626 x 10-34 Js)   c = Speed of Light (3 x 108 m/s)   l = Wavelength (m)   k = Boltzmann Constant (1.38 x 10-23 J/K)  T = Temperature (K)

This complex looking formula is used to plot the black body curves for each temperature by working out the power emitted at each wavelength. A complex formula such as this will give the complex shape of a black body curve. Click here to see more.
 

In the 19th century a major problem for physicists was to predict the intensity of radiation emitted by a black body at a specific wavelength. Wilhelm Wien made a theory that predicted the overall form of the curve by treating the radiation as gas molecules. However, at long wavelengths his theory disagreed with experimental data. Rayleigh and Jeans then produced a formula by considering the radiation within the black body cavity to be made up of a series of standing waves. They thought that electromagnetic radiation was emitted by oscillating atoms in the walls of the black body and this radiation set up a standing wave between the walls.   Their formula stated:
 

I = Intensity

However, this formula also had a problem. For large wavelengths it fitted the experimental data but it had major problems at shorter wavelengths. The problem was the l term in the denominator. It meant that as the wavelength tended to zero, the curve would tend to infinity. However we know that there is a peak wavelength for each temperature, and the energy emitted at either side of this peak dropped. The Rayleigh-Jeans Law predicted no peak wavelength.
 
 

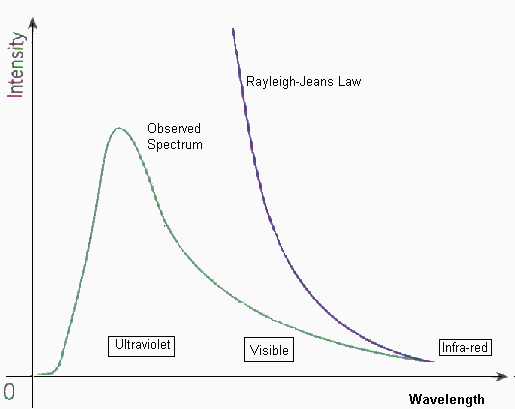Therefore the wave theory of the time could be used to explain behaviour on either side of the peak, but the peak would be infinitely high. The failure of these formulae to account for the decrease in energy emitted at short wavelengths (the ultraviolet wavelengths) became known as the ultraviolet catastrophe. A major breakthrough was made by Max Planck who made a formula that agreed with experimental data, which is showed above. However, he had major problems proving this law. His idea was that the oscillating electrons of the surface atoms of the black body emitted radiation according to Maxwell's laws of electromagnetism. Before Planck it was assumed that these could have any value of energy but Planck decided that the energy must go up in discrete amounts (quantised) because the frequencies of the oscillating electrons could only take certain values. As energy is proportional to frequency (E = hf) , where h is the Planck constant 6.626 x 10^-34 Js) if frequency can only take discrete values, this means that energy is also quantised. The electrons have a fundamental frequency (like standing waves on a string) and the frequency can only go up in whole multiples of this frequency, called the quantum number. This assumption led Planck to correctly derive his formula. If he ignored the quantised energy, he obtained the Rayleigh-Jeans formula. Einstein took the next step by working out that all radiation is quantised. He argued that an oscillating charge can accept or lose energy in small values of DE = hDf. This energy is lost as electromagnetic radiation. Therefore this radiation must be emitted in small packets, each containing DE. He then suggested that each energy of radiation will have its own frequency. Therefore he no longer thought of radiation from an object as continuous. He said it consisted of a series of "packets" of energy. This meant that radiation was being thought of as a "packet of energy" but also as a wave because it had a frequency. These became known as photons.

  This is an effect that is best explained by Einstein's photon model of electromagnetic radiation. Click here to see the equation.When light is shone on metal , the surface may become positively charged. This is because electrons gain energy from the light waves, and are able to leave the metal's surface. However, there were some strange effects that could not be explained by considering light as a wave. The frequency of the light must be above a certain threshold value for that metal for electrons to be emitted. For example blue light causes sodium to emit electrons but red light does not. If light was a wave the electrons would eventually absorb enough energy to be emitted, regardless of frequency. Also, incredibly weak beams of light can cause electrons to be emitted. If light was a wave and spread out you would not expect any electrons to obtain the energy to escape. Finally it was discovered that the kinetic energy of the electrons depends not on the intensity of the light but on its frequency. A very weak ultraviolet beam will give electrons a higher kinetic energy than a very bright blue beam of light. Einstein explained this by saying that the light arrived in photons. One photon gave all its energy to one electron. Click here to see the equation. The electron must gain a certain amount of energy to overcome the forces that hold it inside the metal. It appears that one electron can only accept one photon of energy, so this must have the required frequency to have the necessary escape energy, so the intensity will not make any difference to this. Brighter light means there are more photons, so more electrons can be emitted, but it is the frequency of the light that decides the energy of the photon and so the electron's kinetic energy. Electrons can also be emitted very quickly because it only requires one photon for emission to occur, not the gradual build up of wave energy.